Droplet cloud is an important component of spray, and understanding the combustion mechanism of it benefits in the development of sub-models for spray combustion. Due to the interaction among droplets, droplet clouds tend to burn collectively, i.e. group combustion. The classical droplet group combustion theory was proposed based on the quasi-steady-state assumption, in the framework of which the dimensionless number G was used to classify different combustion modes of droplet clouds - single-droplet combustion, internal group combustion, external group combustion, and sheath combustion. This theory was later validated by steady-state experiments, but was found to be unable to accurately predict the ignition process of droplet clouds due to the quasi-steady-state assumption. In this study, we aim to extend the traditional quasi-steady-state group combustion theory by studying droplet group combustion under transient ignition conditions using detailed numerical simulations.
In this study, we first used numerical simulation to demonstrate that provided the same droplet density, multiple flame structure can exist due to interaction between vaporization, diffusion and chemical kinetics (Figure 1).
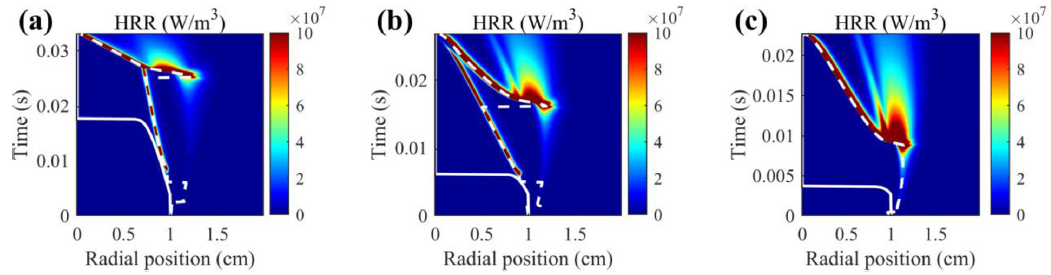
Figure 1 Multi-layered structure for inner gas temperature of 500 K, 700 K and 900 K; the ambient temperature is 1200K
We also compared the ignition modes of droplet clouds with the same G number (Figure 2) and found two regions of uncertain ignition modes (gray areas) outside of the common single-stage ignition and two-stage ignition regions. This indicates that the G number has some predictive power but cannot fully predict the ignition mode of droplet clouds, and this motivates the proposal of a new dimensionless number in this study.

Figure 2 Phase diagram of ignition modes of droplet clouds. The colored solid lines in the figure represent the dividing line of ignition modes at G number consisting of three different subparameters d, n and Rc
After comparing with the classical theory (Figure 3), it is found that the characteristic length scale of droplet cloud combustion in the quasi-steady-state view is the droplet cloud radius Rc, i.e., the effect deeply inside the droplet cloud can be transferred quickly outside the droplet cloud without transient storage; however, in the ignition problem, it is the thin evaporation layer at the edge of the droplet cloud that plays a dominant role, so the characteristic length scale is the vaporization layer thickness Lvp. Based on this knowledge, a new dimensionless number Gig has been derived, Gig = Lvp·(nAd) = 4πn2/3d2. nAd is the total surface area per unit volume of droplets within the thin vaporization layer, and it has a dimension of inverse length scale, which can be physically interpreted as an inverse characteristic length scale of local vaporization.

Figure 3 Illustration of the differences between classical group combustion theory and this work
The newly proposed Gig can successfully predict the ignition modes of droplet clouds (Figure 4), and its temporal value Gig,temp has a strong correlation with the total vaporization rate of droplet cloud. These findings extend the classical droplet cloud combustion theory and provide new ideas for spray combustion modeling.
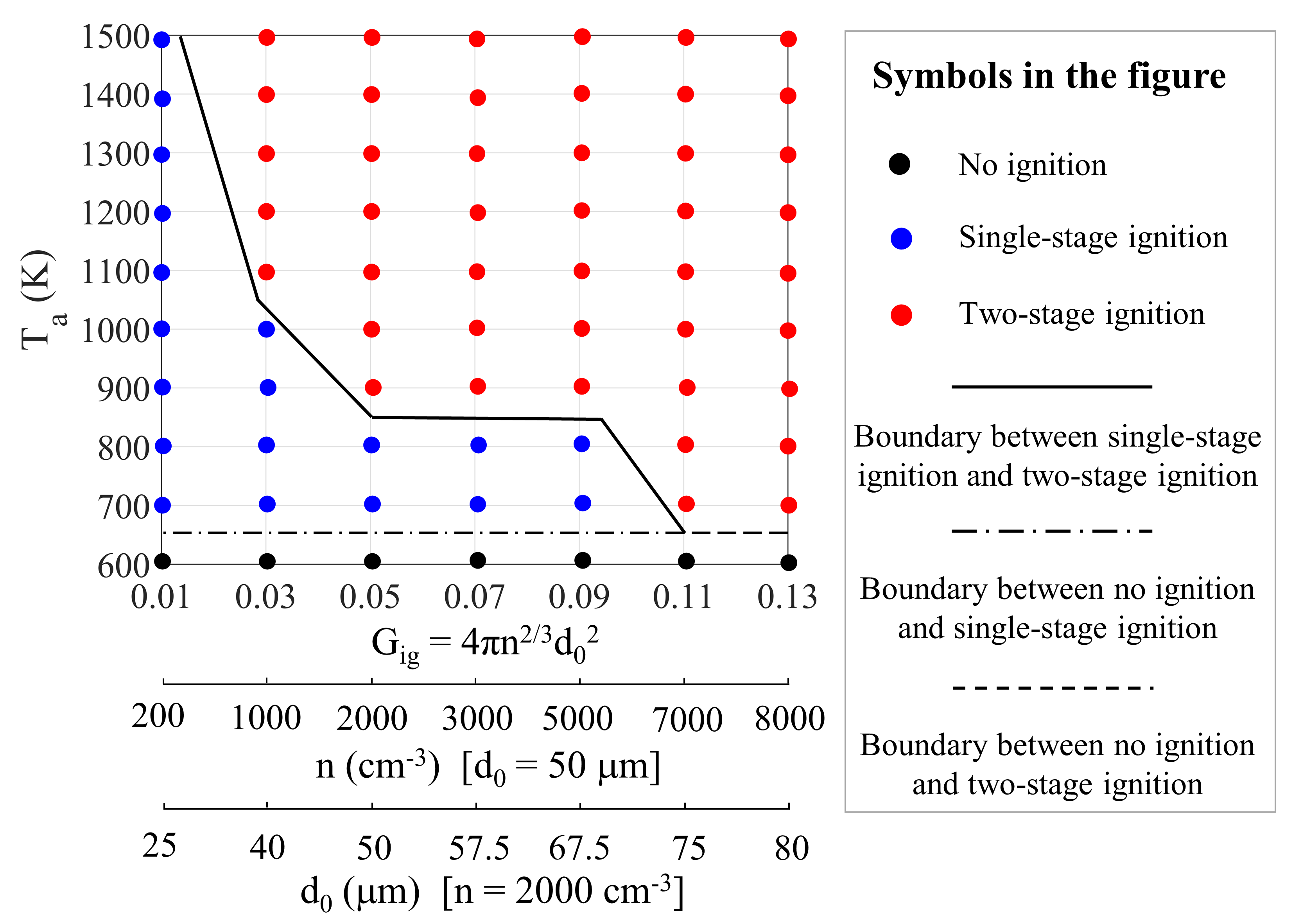
Figure 4 Phase diagram of ignition modes at different ambient temperature Ta and group ignition number Gig
Hengyi Zhou (PhD student, class of 2019) is the first author of this paper, and Prof. Yu Cheng Liu is the corresponding author. This original research article has been published on Combustion and Flame, Aug. 22, 2021.
The link to this paper is: https://doi.org/10.1016/j.combustflame.2021.111689.


